mirror of
https://github.com/chefyuan/algorithm-base.git
synced 2024-11-16 09:03:38 +00:00
85 lines
3.3 KiB
Java
85 lines
3.3 KiB
Java
> 如果阅读时,发现错误,或者动画不可以显示的问题可以添加我微信好友 **[tan45du_one](https://raw.githubusercontent.com/tan45du/tan45du.github.io/master/个人微信.15egrcgqd94w.jpg)** ,备注 github + 题目 + 问题 向我反馈
|
||
>
|
||
> 感谢支持,该仓库会一直维护,希望对各位有一丢丢帮助。
|
||
>
|
||
> 另外希望手机阅读的同学可以来我的 <u>[**公众号:袁厨的算法小屋**](https://raw.githubusercontent.com/tan45du/test/master/微信图片_20210320152235.2pthdebvh1c0.png)</u> 两个平台同步,想要和题友一起刷题,互相监督的同学,可以在我的小屋点击<u>[**刷题小队**](https://raw.githubusercontent.com/tan45du/test/master/微信图片_20210320152235.2pthdebvh1c0.png)</u>进入。
|
||
|
||
#### [209. 长度最小的子数组](https://leetcode-cn.com/problems/minimum-size-subarray-sum/)
|
||
|
||
我们下面再看一种新类型的双指针,也就是我们大家熟知的滑动窗口。这也是我们做题时经常用到的,下面我们来看一下题目吧!
|
||
|
||
#### 题目描述
|
||
|
||
> 给定一个含有 n 个正整数的数组和一个正整数 s ,找出该数组中满足其和 ≥ s 的长度最小的 连续 子数组,并返回其长度。如果不存在符合条件的子数组,返回 0。
|
||
|
||
示例:
|
||
|
||
> 输入:s = 7, nums = [2,3,1,2,4,3]
|
||
> 输出:2
|
||
> 解释:子数组 [4,3] 是该条件下的长度最小的子数组。
|
||
|
||
|
||
|
||
#### 题目解析
|
||
|
||
滑动窗口:**就是通过不断调节子数组的起始位置和终止位置,进而得到我们想要的结果**,滑动窗口也是双指针的一种。
|
||
|
||
下面我们来看一下这道题目的做题思路,其实原理也很简单,我们创建两个指针,一个指针负责在前面探路,并不断累加遍历过的元素的值,当和大于等于我们的目标值时,后指针开始进行移动,判断去除当前值时,是否仍能满足我们的要求,直到不满足时后指针 停止,前面指针继续移动,直到遍历结束。是不是很简单呀。前指针和后指针之间的元素个数就是我们的滑动窗口的窗口大小。见下图
|
||
|
||
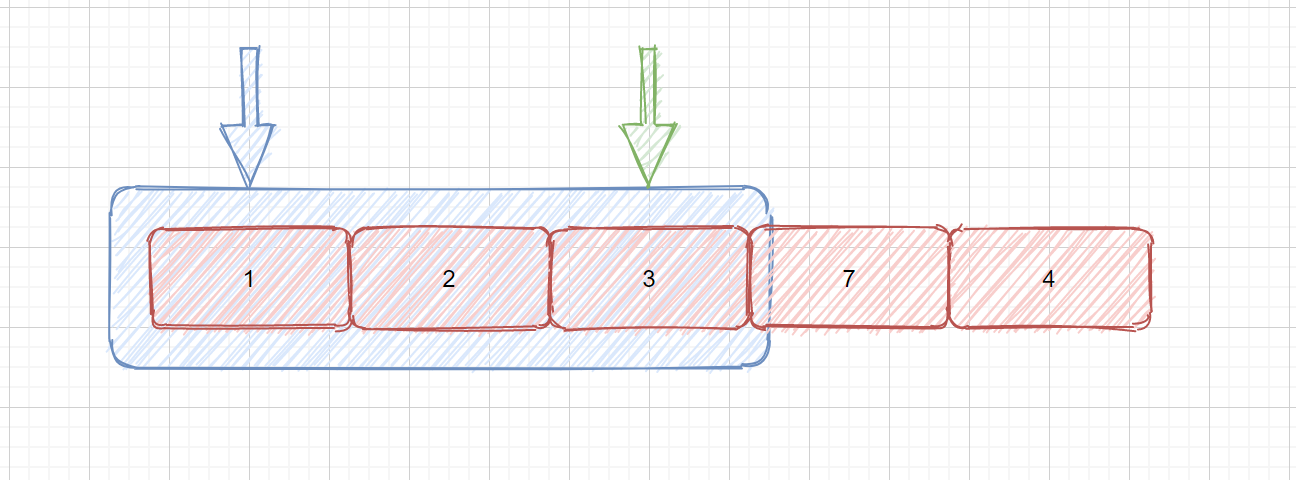
|
||
|
||
|
||
|
||
好啦,该题的解题思路我们已经了解啦,下面我们看一下,代码的运行过程吧。
|
||
|
||
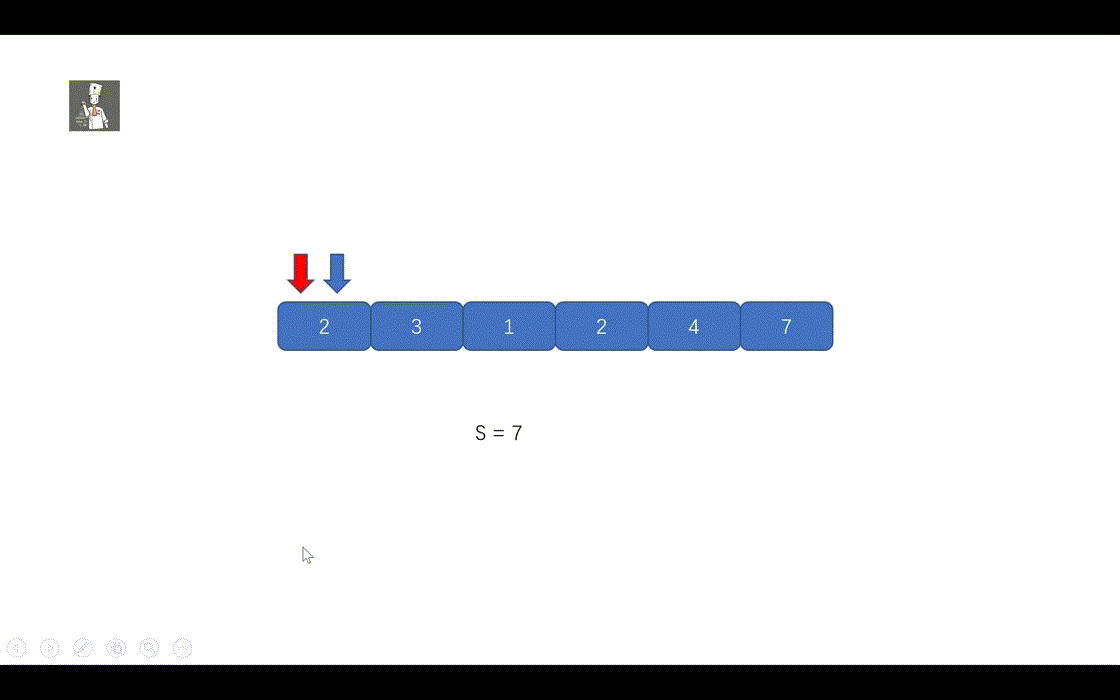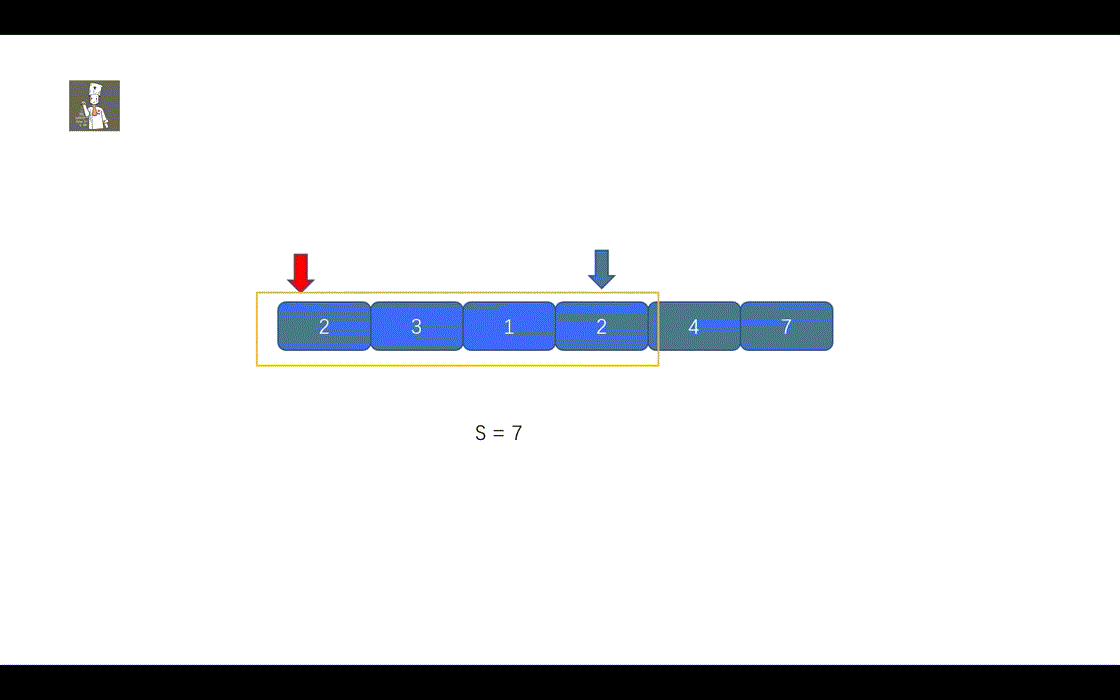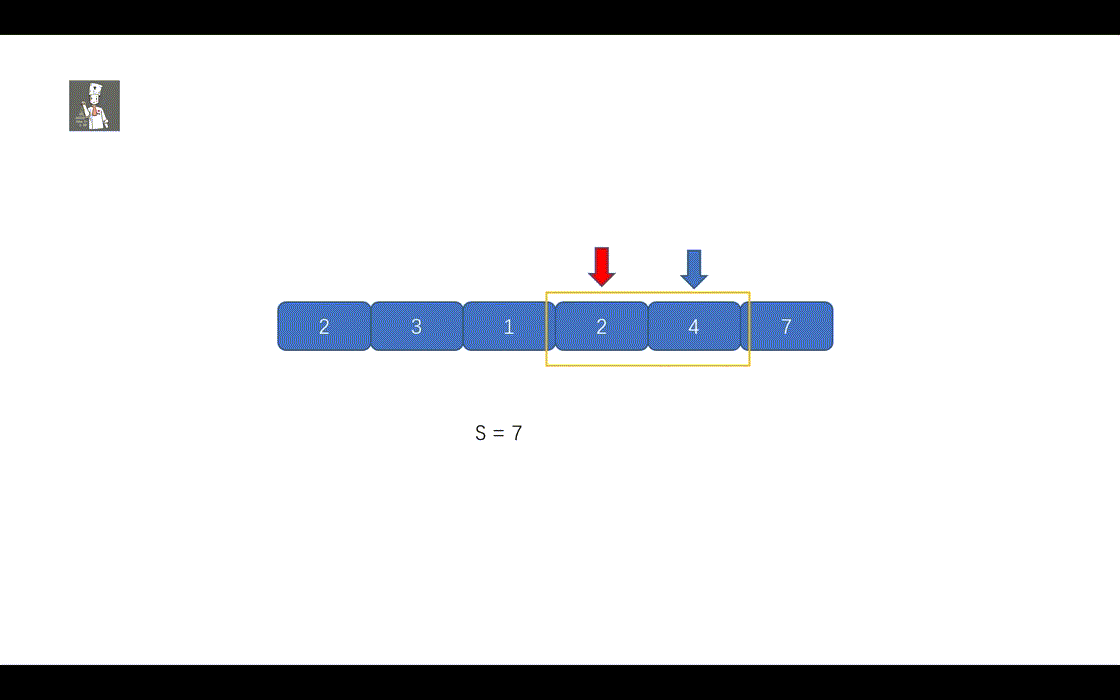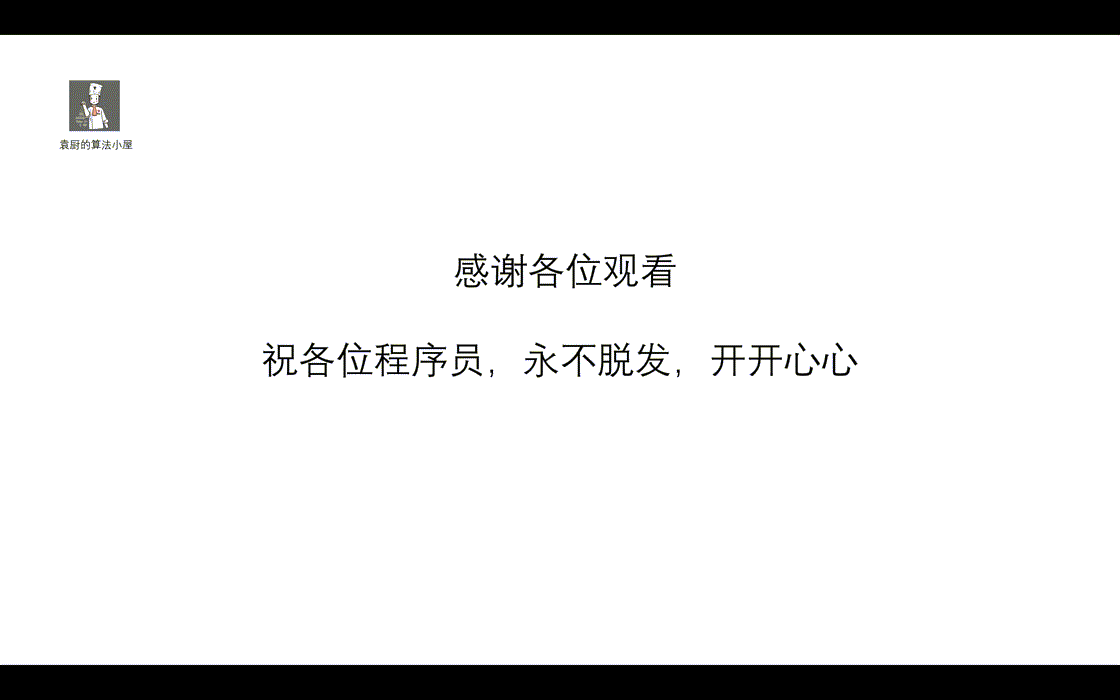
|
||
|
||
|
||
|
||
#### 题目代码
|
||
|
||
Java Code:
|
||
|
||
```java
|
||
class Solution {
|
||
public int minSubArrayLen(int s, int[] nums) {
|
||
|
||
int len = nums.length;
|
||
int windowlen = Integer.MAX_VALUE;
|
||
int i = 0;
|
||
int sum = 0;
|
||
for (int j = 0; j < len; ++j) {
|
||
sum += nums[j];
|
||
while (sum >= s) {
|
||
windowlen = Math.min (windowlen, j - i + 1);
|
||
sum -= nums[i];
|
||
i++;
|
||
}
|
||
}
|
||
return windowlen == Integer.MAX_VALUE ? 0 : windowlen;
|
||
|
||
}
|
||
}
|
||
```
|
||
|
||
C++ Code:
|
||
|
||
```cpp
|
||
class Solution {
|
||
public:
|
||
int minSubArrayLen(int t, vector<int>& nums) {
|
||
int n = nums.size();
|
||
int i = 0, sum = 0, winlen = INT_MAX;
|
||
for(int j = 0; j < n; ++j){
|
||
sum += nums[j];
|
||
while(sum >= t){
|
||
winlen = min(winlen, j - i + 1);
|
||
sum -= nums[i++];
|
||
}
|
||
}
|
||
return winlen == INT_MAX? 0: winlen;
|
||
}
|
||
};
|
||
```
|
||
|